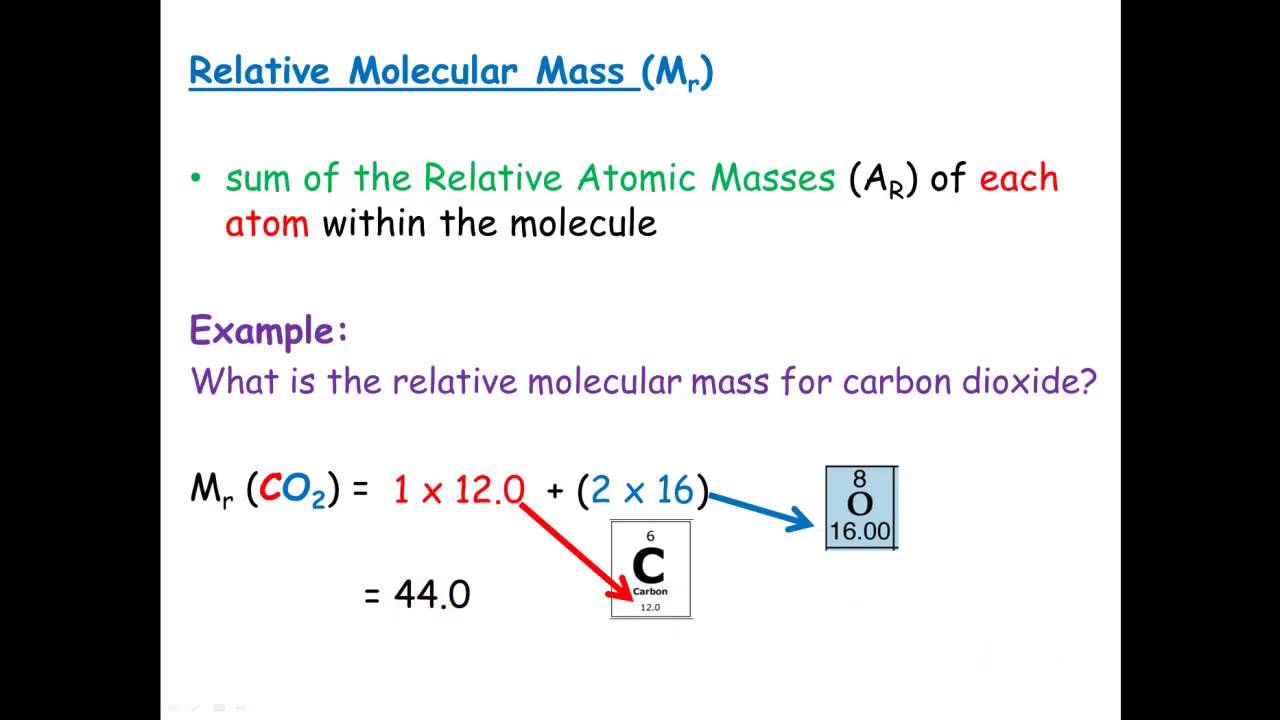
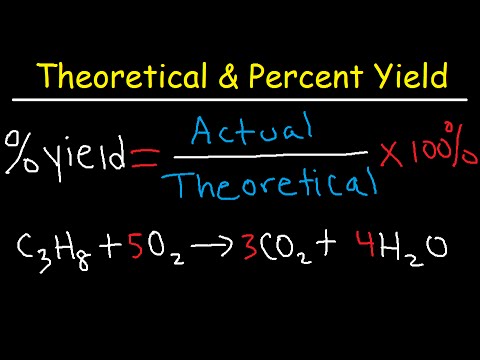
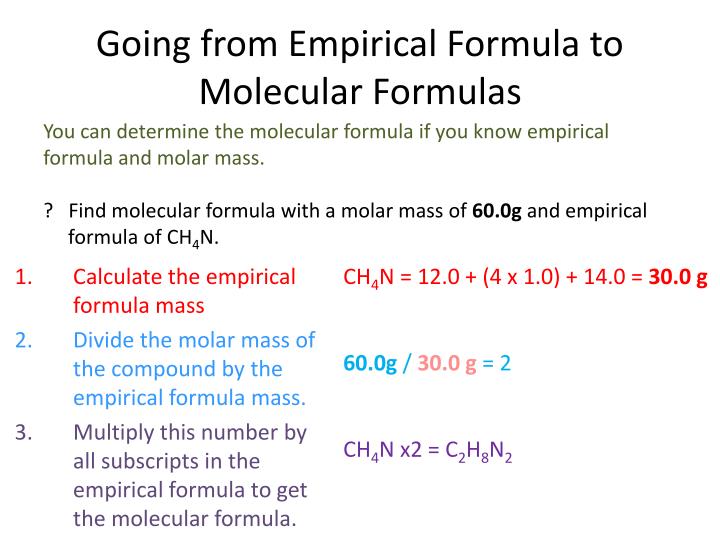
Volume is an amount of space, in three dimensions, that a sample of matter occupies. The number and the phase of the molecules in the sample primarily determine the volume of a substance. Volume will be measured in many ways in this course, but the units are usually milliliters or cubic centimeters . Methods for determining or delivering precise volumes include volumetric pipets and pycnometers; less precise methods include burets, graduated cylinders, and graduated pipets. What two things do you need to know in order to find the density of water?
Students should realize that they need both the volume and mass of a sample of water to find its density. Suggest that students use a graduated cylinder to measure volume in milliliters. Remind students that each milliliter equals 1 cm3.
Suggest that students use a balance to measure the mass in grams. Tell students that they can find mass by weighing the water. However, since water is a liquid, it needs to be in some sort of container. So in order to weigh the water, they have to weigh the container, too.
Explain to students that they will have to subtract the mass of an empty graduated cylinder from the mass of the cylinder and water to get the mass of just the water. Volume is the quantification of the three-dimensional space a substance occupies. The SI unit for volume is the cubic meter, or m3. By convention, the volume of a container is typically its capacity, and how much fluid it is able to hold, rather than the amount of space that the actual container displaces. Volumes of many shapes can be calculated by using well-defined formulas.
In some cases, more complicated shapes can be broken down into simpler aggregate shapes, and the sum of their volumes is used to determine total volume. The volumes of other even more complicated shapes can be calculated using integral calculus if a formula exists for the shape's boundary. Beyond this, shapes that cannot be described by known equations can be estimated using mathematical methods, such as the finite element method. Alternatively, if the density of a substance is known, and is uniform, the volume can be calculated using its weight. This calculator computes volumes for some of the most common simple shapes. The new platforms, a Sophion Biosciences Qube 384 MkII automated electrophysiology instrument will complement the Company's three existing, high fidelity QPatch 48 channel systems.
The Molecular Devices 384-well FLIPR® Penta enables fluorescence-based HTS hit finding and medicinal chemistry triage. Both systems will also provide support to the Metrion's expanded cell biology activities. The density of a substance can be used to define the substance.Water is unusual because when water freezes, its solid form is less dense than liquid water, and thus floats on top of liquid water. Pour the tinted water into the 500-mL volumetric flask, using a funnel if needed. Pour the tinted ethyl alcohol into the 500-mL volumetric flask, using a funnel if needed.
Note the level of the liquid in the 500-mL volumetric flask. (It should be very close to the 500-mL volume line.) Place a stopper or cap on the 500-mL volumetric flask and invert the flask several times. Remove the stopper or cap to prevent a vacuum from occurring inside flask.
Replace the cover and continue mixing the solution by inverting the flask until a green color is consistent throughout the solution. Allow the solution to settle, then observe the volume of liquid. (It should be far below the 500-mL volume line.) Mass the 500-mL volumetric flask containing the water-alcohol solution. Calculate the mass of the water-alcohol solution. Determine how much volume was lost in the creation of this solution.
Using a disposable pipet, add water from a graduated cylinder to the volumetric flask containing the water-alcohol solution. Subtract this volume from 500 mL to obtain the final volume. Because gases fill their containers, their volume is the same as the internal volume of the container.
Liquids are commonly measured using containers, where the volume is marked or else is the internal shape of the container. Examples of instruments used to measure liquid volume include measuring cups, graduated cylinders, flasks, and beakers. There are formulas for calculating the volume of regular solid shapes. Another method of determining the volume of a solid is to measure how much liquid it displaces. Calculate the uncertainty in the mass of water removed using error propagation.
Convert this mass to volume units by dividing by the density of water (use a precise value, specific to the water's temperature). This value equals the uncertainity in the volume of the metal cylinder. To use the water displacement method, an object is inserted into a graduated cylinder partially filled with water.
The object's volume occupies space, displacing liquid and raising the water level. The difference between the two volumes, before and after the object was inserted, is the object's volume. To calculate the mass of an object, look up the recorded density of the object online or in a textbook, which will be in units of kg/m3 or g/cm3. Then, multiply the density of the object by it's measured volume.
Make sure that your measurements for volume and density are in the same units! For example, if you have a diamond with a volume of 5,000 cm3 and density of 3.52 g/cm3, multiply 5,000 cm3 by 3.52 g/cm3 to get the mass of 17,600 grams. In the newly created position of Head of Screening Technologies, Dr Gary Clark will lead the implementation of the expanded services as well as the company's enhanced cell biology capabilities. Look at your values for density in your chart.
Does the density of the different volumes of water seem to be about the same? Help students see that most of the different values for density are near 1 g/cm3. They may wonder why their values are not all exactly 1 g/cm3.
One reason could be inaccuracies in measuring. Another reason is that the density of water changes with temperature. Water is most dense at 4 °C and at that temperature has a density of 1 g/cm3. At room temperature, around 20–25 °C, the density is about 0.99 g/cm3.
Students answers will vary, but their values should mostly be around 1 g/cm3. Each group measured the volume of the same samples. What are some reasons that groups might have different values for density? Students should realize that small inaccuracies in measuring volume can account for differences in density values. Another reason is that the graduated cylinder, itself, is not perfect. So there is always some uncertainty in measuring.
Remind students that in the beginning of the lesson they made a prediction about the density of the small, medium, and long sample. Students should have predicted that the longest cylinder has the lowest density, the shortest cylinder has the highest density, and the middle is somewhere in between. It's usually fairly easy to calculate the volume of a liquid in a container with a regular shape, such as a cylinder or cube. All you have to do is use the appropriate mathematical equation to calculate the capacity of the container, then measure the level of the liquid and make the necessary adjustment. It's more challenging when the container doesn't have a regular shape, and that's most of them.
The challenge disappears if you know the density of the liquid, though. All you have to do is weigh the container and the liquid, subtract the weight of the container, and use the density of the liquid to derive your answer. Scientists who work in a laboratory must be familiar with typical laboratory glassware, often called volumetric glassware. These may include beakers, a volumetric flask, an Erlenmeyer flask, and a graduated cylinder. Each of these containers is used in a laboratory setting to measure liquid volumes for different purposes.
This work presents the characterization of N,N'-diacetyl-1,13-diaza-24-crown-8 by quantum chemical calculations and spectral techniques. The calculated results show that the optimized geometry can well reproduce the crystal structure, and the theoretical chemical shift values show good agreement with experimental values. The predicted non-linear optical properties of I are greater than ones of urea. In addition, DFT calculations of molecular electrostatic potentials and frontier molecular orbitals of I were carried out at the B3LYP/6â'31G level of theory. Start the demonstration by asking students to predict what will occur when an equal volume of water and ethyl alcohol are mixed. Specifically, ask them if they think the mass and volume will be additive.
The following table is the answer key to the Water-Alcohol Demonstration Worksheet located at the end of this activity. During the demonstration, students record data to their worksheet table. This demonstration is best performed using 3 volumetric flasks, which provide a good opportunity to instruct students in the purpose and use of volumetric flasks. (However, you can use whatever glassware is available.) Using volumetric flasks makes this a very quantitative demonstration. The larger the volumetric flask, the easier it is for students to observe the difference in volume. A lower-cost alternative to volumetric flasks is using 3 graduated cylinders.
A 3rd alternative is using a large-diameter tube (e.g., 10 to 25 mm inside diameter) with 2 rubber stoppers to seal each end. With this alternative, a vapor bubble appears as the solution is mixed and the volume reduces. Mass is one of the fundamental properties of an object in Physics, and is a measurement of how much matter there is in something. Matter is any substance that you can touch — anything that takes up physical space and has volume. Often, mass is related to size, but this isn't a perfect relationship, as objects like a large hot-air balloon often have less mass than a small boulder.
To calculate mass, you'll first need the density and volume of the object. Read on for details of the formula and to learn about different types of mass across scientific disciplines. If you want to know how to find density, keep reading. This article will provide you with the density formula, which this calculator is based on. You'll also learn how the density of water changes under different circumstances.
To perform pycnometry measurements, the mass of the cylinder and the mass of a flask filled with water to a mark (A, Fig. 3) are recorded. Water is displaced when the cylinder is inserted. The volume of water displaced is removed by pipet, thereby restoring the water level to the mark .
The combined mass of the flask, remaining water, and cylinder is then measured. For less defined shapes, volume can be determined by water displacement. Volumes of liquids such as water can be readily measured in a graduated cylinder.
Students will be able to measure the volume and mass of water and calculate its density. Students will be able to explain that since any volume of water always has the same density, at a given temperature, that density is a characteristic property of water. Students measure the volume and mass of water to determine its density. Then they measure the mass of different volumes of water and discover that the density is always the same.
Students make a graph of the relationship between the volume and the mass of water. Students use the water displacement method to find the volume of different rods that all have the same mass. They calculate the density of each rod, and use the characteristic density of each material to identify all five rods. Then students consider the relationship between the mass, size, and arrangement of atoms to explain why different rods have different densities.
Students will be briefly introduced to the periodic table. You determine the mass of a substance by weighing it. This can cause some confusion, because weight and mass are different quantities. Mass is a measure of the amount of matter whereas weight is a measure of the force of gravity.
However, it's common to use kilograms, grams or pounds for both weight and mass, and this is because, for earthbound objects, the relationship between mass and weight doesn't change. This isn't true for objects in space, but few scientists have the opportunity to make measurements in space. For all 3 options, the volume of each liquid—water and ethyl alcohol—should be ½ the total volume of the final solution. Use food coloring to provide a visual indicator of the mixed solution.
If needed, add a piece of black electrical tape at the volume line of each volumetric flask to assist students with their visual observation. Convert this mass to volume by dividing by the density of water (use a precise value, specific to the water's temperature). This volume equals the volume of the metal cylinder. Play the animation as you demonstrate the water displacement method using a cup of water, a graduated cylinder, and a rod, the way students will do in the activity. Use the dark gray plastic sample so that students can see it better. Tell students that like the cubes in the previous activity, they will need to know the volume and mass of each of the samples.
They will also calculate the density of each sample and use this value to figure out which material each rod is made of. Pour the fluid into a graduated cylinder and read its volume. The weight of the fluid is the difference between the two weighings. Note that this method, after pouring the fluid from the graduated cylinder, includes the remnants of fluid in the volume reading, but not in the mass calculation. Firstly it is important to understand that when you're looking at the chemistry of solutions there are endless factors to consider.